The Science Behind Everything
One place for all scientific and technical information. Paradox, Multiverse, Relativity, Speed.
Thursday, 27 July 2017
Saturday, 28 January 2017
DC Multiverse
The Multiverse, within DC Comics publications, is a "cosmic construct" collecting many of the fictional universes in which the published stories take place. The worlds in this multiverse share a space and fate in common and its structure has changed several times in the history of DC Comics.
DC comics showcases multiple universes in alternate timelines each occupying the same space. But one might ask how can there be infinite universes all occupying the same space?
DC Comics answers it by telling that all the universes vibrate at different frequencies hence enabling them to occupy the same space.
There are various multiverses by DC comics but I am going to elaborate only the recent one, i.e. The New 52 in this post. To all the readers, if you want to know more about the other DC multiverses, please leave a comment and I'll post on it soon.
An infinitely powerful and god-like version of Brainiac abducted multiple superheroes and their core cities from various alternate Earths and eras of the Multiverse and pitted them against one another. Because of the heroes efforts for the survival of reality during this event, the events of Crisis on Infinite Earths and Zero Hour were averted and there is once more an infinite Multiverse beyond the core 52 universes which have made up the local Multiverse since Infinite Crisis and Flashpoint. The original Multiverse coexists along with the collapsed Earth, the core 52 worlds, and other versions of the universe that had ever existed.
DC comics showcases multiple universes in alternate timelines each occupying the same space. But one might ask how can there be infinite universes all occupying the same space?
DC Comics answers it by telling that all the universes vibrate at different frequencies hence enabling them to occupy the same space.
There are various multiverses by DC comics but I am going to elaborate only the recent one, i.e. The New 52 in this post. To all the readers, if you want to know more about the other DC multiverses, please leave a comment and I'll post on it soon.
The New 52
The Flash wakes up in an altered timeline. As he tries to find the cause, he discovers that he was responsible for the alteration and attempts to fix it. In doing so, it is revealed that the timelines of Earth-0, Earth-13 and Earth-50 were originally one, but were splintered. The result is a new timeline formed by those three and along with it came a new history for the other 52 worlds within the Multiverse (Flashpoint, 2011). It is later revealed that in actuality, the current timeline was created when a mysterious being (implied to be Doctor Manhattan from Watchmen) entered the Multiverse while the timeline was resetting due to Barry Allen preventing the Flashpoint event, and extracted ten years from continuity (DC Universe: Rebirth, 2016)
Years later, The Harbinger Program at the House of Heroes gathers several heroes of the "Orrery" to fight against a force known as the "Gentry" who have already decimated Earth-7 and threaten the rest of the worlds of the Multiverse. As the story unfolds, Earths within the Orrery are visited and reveal the new nature of them after the Flashpoint event. Also, mysterious comic books published by DC and Major Comics appear and are believed to be cursed or to be messages from parallel earths (The Multiversity, 2014).
Several stories and even the structure of the entire Multiverse have been retold after the events of Flashpoint. As it has been revealed so far, most of the 52 worlds suffered drastic changes such as Earth-2 which is now a reboot in the present day of the heroes that formed the Justice Society or Earth-3 which reverted to be the opposite of the main Earth (Earth-0 in this case), instead of the opposite of Earth-2. Others retain most of what they were in the 52 multiverse such as Earth-5, Earth-10, or Earth-23. In addition, several Earths remain undisclosed in composition or purpose, other than their creation by the Monitors for unknown reasons- Earths 14, 24, 25, 27, 28, 46, and 49.
The Monitors are now described as a race of countless members and only 52 remained after the CRISIS event, suggesting that there were Monitors for every world in the original Multiverse instead of just one. Several elements that have appeared across the history to what now is DC Comics have also been actively incorporated in the new structure, such as The Source (The New Gods), The Bleed (Wildstorm's The Authority), the Speed Force and the vibrational barriers (The Flash) and the Rock of Eternity (SHAZAM!).
This new Multiverse has a sphere-like structure with several levels (or Vibrational Realms) as described in the map:
- The Source Wall: the limit of existence, beyond lies the Source and the Unknowable. The Overvoid is shown in the map to exist outside it as well.
- Monitor Sphere: origin of the Monitor race who preserve and study the universes.
- Limbo: "where matter and memory break down". Place where the lost and forgotten go.
- Sphere of the Gods: within it, the realms of old and new gods, demons and even dreams exist.
- Speed Force Wall: also known as the Speed of Light and is the limit to matter. Within it is the Orrery of Worlds and certain worlds exist in it (such as Krakkl's world).
- Orrery of Worlds: realm where the 52 universes exist in the same space, vibrating at different frequencies, within the Bleed. In the center of it are the Rock of Eternity and theHouse of Heroes.
An infinitely powerful and god-like version of Brainiac abducted multiple superheroes and their core cities from various alternate Earths and eras of the Multiverse and pitted them against one another. Because of the heroes efforts for the survival of reality during this event, the events of Crisis on Infinite Earths and Zero Hour were averted and there is once more an infinite Multiverse beyond the core 52 universes which have made up the local Multiverse since Infinite Crisis and Flashpoint. The original Multiverse coexists along with the collapsed Earth, the core 52 worlds, and other versions of the universe that had ever existed.
Dark Matter
Dark matter is an unidentified type of matter distinct from dark energy, baryonic matter (ordinary matter), and neutrinos. The name refers to the fact that it does not emit or interact with electromagnetic radiation, such as light, and is thus invisible to the entire electromagnetic spectrum. Although dark matter has not been directly observed, its existence and properties are inferred from its gravitational effects such as the motions of visible matter, gravitational lensing, its influence on the universe's large-scale structure, on galaxies, and its effects in the cosmic microwave background.
The standard model of cosmology indicates that the total mass–energy of the universe contains 4.9% ordinary matter, 26.8% dark matter and 68.3% dark energy. Thus, dark matter constitutes 84.5% of total mass, while dark energy plus dark matter constitute 95.1% of total mass–energy content. The great majority of ordinary matter in the universe is also unseen, since visible stars and gas inside galaxies and clusters account for less than 10% of the ordinary matter contribution to the mass-energy density of the universe. The most widely accepted hypothesis on the form for dark matter is that it is composed of weakly interacting massive particles (WIMPs) that interact only through gravity and the weak force.
The dark matter hypothesis plays a central role in current modeling of cosmic structure formation and galaxy formation and evolution and on explanations of the anisotropies observed in the cosmic microwave background (CMB). All these lines of evidence suggest that galaxies, galaxy clusters, and the universe as a whole contain far more matter than that which is observable via electromagnetic signals. Many experiments to detect proposed dark matter particles through non-gravitational means are under way; however, no dark matter particle has been conclusively identified.
Although the existence of dark matter is generally accepted by most of the astronomical community, a minority of astronomers, motivated by the lack of conclusive identification of dark matter, argue for various modifications of the standard laws of general relativity, such as MOND, TeVeS, and conformal gravity that attempt to account for the observations without invoking additional matter.
Observational evidence
Much of the evidence comes from the motions of galaxies. Many of these appear to be fairly uniform, so by the virial theorem, the total kinetic energy should be half the galaxies' total gravitational binding energy. Observationally, the total kinetic energy is much greater. In particular, assuming the gravitational mass is due to only visible matter, stars far from the center of galaxies have much higher velocities than predicted by the virial theorem. Galactic rotation curves, which illustrate the velocity of rotation versus the distance from the galactic center, show the "excess" velocity. Dark matter is the most straightforward way of accounting for this discrepancy.
The distribution of dark matter in galaxies required to explain the motion of the observed matter suggests the presence of a roughly spherically symmetric, centrally concentrated halo of dark matter with the visible matter concentrated in a central disc.
Low surface brightness dwarf galaxies are important sources of information for studying dark matter. They have an uncommonly low ratio of visible to dark matter, and have few bright stars at the center that would otherwise impair observations of the rotation curve of outlying stars.
Gravitational lensing observations of galaxy clusters allow direct estimates of the gravitational mass based on its effect on light coming from background galaxies, since large collections of matter (dark or otherwise) gravitationally deflect light. In clusters such as Abell 1689, lensing observations confirm the presence of considerably more mass than is indicated by the clusters' light. In the Bullet Cluster, lensing observations show that much of the lensing mass is separated from the X-ray-emitting baryonic mass. In July 2012, lensing observations were used to identify a "filament" of dark matter between two clusters of galaxies, as cosmological simulations predicted.
In August 2016, astronomers reported that Dragonfly 44, an ultra diffuse galaxy (UDG) with the mass of the Milky Way galaxy, but with nearly no discernable stars or galactic structure, may be made almost entirely of dark matter.
Wednesday, 25 January 2017
Speed !
The highest speed achieved by man is interestingly 24,791miles per hour, i.e. 89247.6 kilometres per hour and about 6886.3889 meters per second. When it comes to vehicles built for space travel, the speed record is staggering. Upon re-entry to the Earth's atmosphere on May 26, 1969, the Apollo 10 Lunar Module reached speeds of 24,791 miles per hour.
But can we travel at the speed of light?
Light, according to Maxwell, was a vibration in the electromagnetic field and it travelled at a constant speed in a vacuum. More than 100 years earlier, Newton had set down his laws of motion and, together with ideas from Galileo Galilei, these showed how the speed of an object would differ depend on who was measuring it and how they were moving relative to the object. A ball you are holding will seem still to you, even when you're in a moving car. But that ball will seem to be moving to anyone standing on the pavement.
But there was a problem in applying Newton's laws of motion to light. In Maxwell's equations, the speed of electromagnetic waves is a constant defined by the properties of the material through which the waves move. There is nothing in there that allows the speed of these waves to be different for different people depending on how they were moving relative to each other. Which is bizarre, if you think about it.
Imagine someone sitting in a stationary train, throwing a ball from where he's sitting to the opposite wall, a few metres further down the train from him. You, standing on the station platform, measure the speed of the ball at the same value as the person on the train.
Now the train starts to move (in the direction of the ball), and you again measure the speed of the ball. You would rightly calculate it as higher – the initial speed (ie, when the train was at rest) plus the forward speed of the train. On the train, meanwhile, the game-player will notice nothing different. Your two values for the speed of the ball will be different; both correct for your frames of reference.
Replace the ball with light and this calculation goes awry. If the person on the train were shining a light at the opposite wall and measured the speed of the particles of light (photons), you and the passenger would both find that the photons had the same speed at all times. In all cases, the speed of the photons would stay at just under 300,000 kilometres per second, as Maxwell's equations say they should.
Einstein took this idea – the invariance of the speed of light – as one of his two postulates for the special theory of relativity. The other postulate was that the laws of physics are the same wherever you are, whether on an plane or standing on a country road. But to keep the speed of light constant at all times and for all observers, in special relativity, space and time become stretchy and variable. Time is not absolute, for example. A moving clock ticks more slowly than a stationary one. Travel at the speed of light and, theoretically, the clock would stop altogether.
How much the time dilates can be calculated by the two equations above. On the right, Δt is the time interval between two events as measured by the person they affect. (In our example above, this would be the person in the train.) On the left, Δt' is the time interval between the same two events but measured by an outside observer in a separate frame of reference (the person on the platform). These two times are related by the Lorentz factor (γ), which in this example is a term that takes into account the velocity (v) of the train relative to the station platform, which is "at rest". In this expression, c is a constant equal to the speed of light in a vacuum.
The length of moving objects also shrink in the direction in which they move. Get to the speed of light (not really possible, but imagine if you could for a moment) and the object's length would shrink to zero.
The contracted length of a moving object relative to a stationary one can be calculated by dividing the proper length by the Lorentz factor – if it were possible for an object to reach the speed of light its length would shrink to zero.
It is important to note that if you were the person moving faster and faster, you would not notice anything: time would tick normally for you and you would not be squashed in length. But anyone watching you from the celestial station platform would be able to measure the differences, as calculated from the Lorentz factor. However, for everyday objects and everyday speeds, the Lorentz factor will be close to 1 – it is only at speeds close to that of light that the relativistic effects need serious attention.
Another feature that emerges from special relativity is that, as something speeds up, its mass increases compared with its mass at rest, with the mass of the moving object determined by multiplying its rest mass by the Lorentz factor. This increase in relativistic mass makes every extra unit of energy you put into speeding up the object less effective at making it actually move faster.
As the speed of the object increases and starts to reach appreciable fractions of the speed of light (c), the portion of energy going into making the object more massive gets bigger and bigger.
This explains why nothing can travel faster than light – at or near light speed, any extra energy you put into an object does not make it move faster but just increases its mass. Mass and energy are the same thing – this is a profoundly important result. But that is another story.
Tuesday, 24 January 2017
What is Einstien's so famous equation in mathematics?
Einstein's theory of special relativity is based on two assumptions:
Imagine a spacecraft passing the Earth with velocity v. On the spacecraft is an observer and an apparatus that will flash a beam of light across the spacecraft (perpendicular to the spacecraft's motion). On the Earth is another observer.
According to the observer on the spacecraft, the light beam travels a distance w, where w is the width of the spacecraft. However, the observer of Earth will see the light beam cover a greater distance, due to the motion of the spacecraft while the light beam is en route. If t is the time the light beam takes to cross the spacecraft, then the spacecraft travels distance vt in this time. Then the distance travelled by the light beam is

Now in pre-relativistic (Newtonian) physics, both observers record the same period of time. Thus, the velocity recorded by the two observers is different: the Earth-bound observer would record a greater velocity for the beam of light.
However, Einstein's assumption is that the velocity of light is the same for both observers.
The speed of light, c, would be calculated by the observer on the spacecraft as:


Here, d' and t' are distance and time, respectively, measured on the spacecraft.
Solving for t' gives:

The speed of light calculated by the observer on Earth is:
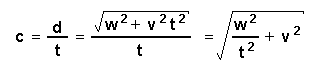
Solving for t gives:

To find the ratio of time measured on the spacecraft to time measured on Earth, find t'/t:

If v << c, then the result t'/t is very close to 1. But as v approaches c, t'/t becomes smaller. Time slows down for the observer on the spacecraft as its speed approaches the speed of light!
The speed of light in vaccum (c) is 299,792.458 kilometers/second. Because c is so much larger than the speeds encountered in human experience (thus far!) the resultant time dilation is negligible. The fastest speed ever attained by humans (relative to the Earth) was 11.1 kilometers/second, by Apollo 10 astronauts returning from the Moon in 1969. For them, t'/t = 0.9999999993. This is equivalent to losing 1 second in 45 years.
At speeds on the order of the speed of light, this time dilation is significant. If v/c = 0.6, then t'/t = 0.8. If v/c = 0.99, then t'/t = 0.141.
Comparable transformations apply to the length of an object along the direction of travel and the object's mass:

Here, p' is the relativistic momentum and m is the rest mass.
This has some interesting consequences. First, for an object with non-zero mass as its velocity approaches the speed of light its momentum will approach infinity. This implies that that it would take an infinite amount of energy to accelerate an object with mass to the speed of light. Since this amount of energy is unavailable, objects with mass cannot travel at the speed of light (in vaccum) or faster. General relativity will expand on this understanding of the speed of light as a limiting speed in the universe.
Now we can show something else regarding the mass change. If a or b are (positive) numbers much less than one, then these approximations apply:

If the speed is much smaller then the speed of light, or v << c, then we can apply these approximations to the above formula for m'/m and get:

If we multiply both sides by c², the result is:

Notice that the second term on the right is the kinetic energy in pre-relativistic physics. The equation thus states that some amount of energy (mc²) plus kinetic energy gives what we can call the relativistic energy of an object. Clearly, an object that is not moving has an associated amount of energy

This is Einstein's famous equation relating mass and energy. Special relativity concludes that mass and energy are equivalent concepts.
- All inertial (i.e. non-accelerating) frames of reference are equally valid (i.e. any observations or experiments performed will produce equally valid results).
- The speed of light is constant for all inertial frames of reference.
Imagine a spacecraft passing the Earth with velocity v. On the spacecraft is an observer and an apparatus that will flash a beam of light across the spacecraft (perpendicular to the spacecraft's motion). On the Earth is another observer.
According to the observer on the spacecraft, the light beam travels a distance w, where w is the width of the spacecraft. However, the observer of Earth will see the light beam cover a greater distance, due to the motion of the spacecraft while the light beam is en route. If t is the time the light beam takes to cross the spacecraft, then the spacecraft travels distance vt in this time. Then the distance travelled by the light beam is
Now in pre-relativistic (Newtonian) physics, both observers record the same period of time. Thus, the velocity recorded by the two observers is different: the Earth-bound observer would record a greater velocity for the beam of light.
However, Einstein's assumption is that the velocity of light is the same for both observers.
The speed of light, c, would be calculated by the observer on the spacecraft as:

Here, d' and t' are distance and time, respectively, measured on the spacecraft.
Solving for t' gives:
The speed of light calculated by the observer on Earth is:
Solving for t gives:
To find the ratio of time measured on the spacecraft to time measured on Earth, find t'/t:
If v << c, then the result t'/t is very close to 1. But as v approaches c, t'/t becomes smaller. Time slows down for the observer on the spacecraft as its speed approaches the speed of light!
The speed of light in vaccum (c) is 299,792.458 kilometers/second. Because c is so much larger than the speeds encountered in human experience (thus far!) the resultant time dilation is negligible. The fastest speed ever attained by humans (relative to the Earth) was 11.1 kilometers/second, by Apollo 10 astronauts returning from the Moon in 1969. For them, t'/t = 0.9999999993. This is equivalent to losing 1 second in 45 years.
At speeds on the order of the speed of light, this time dilation is significant. If v/c = 0.6, then t'/t = 0.8. If v/c = 0.99, then t'/t = 0.141.
Comparable transformations apply to the length of an object along the direction of travel and the object's mass:
Here, p' is the relativistic momentum and m is the rest mass.
This has some interesting consequences. First, for an object with non-zero mass as its velocity approaches the speed of light its momentum will approach infinity. This implies that that it would take an infinite amount of energy to accelerate an object with mass to the speed of light. Since this amount of energy is unavailable, objects with mass cannot travel at the speed of light (in vaccum) or faster. General relativity will expand on this understanding of the speed of light as a limiting speed in the universe.
Now we can show something else regarding the mass change. If a or b are (positive) numbers much less than one, then these approximations apply:
If the speed is much smaller then the speed of light, or v << c, then we can apply these approximations to the above formula for m'/m and get:
If we multiply both sides by c², the result is:
Notice that the second term on the right is the kinetic energy in pre-relativistic physics. The equation thus states that some amount of energy (mc²) plus kinetic energy gives what we can call the relativistic energy of an object. Clearly, an object that is not moving has an associated amount of energy
This is Einstein's famous equation relating mass and energy. Special relativity concludes that mass and energy are equivalent concepts.
Theory of Relativity
The theory of relativity usually encompasses two interrelated theories by Albert Einstein: special relativity and general relativity. Special relativity applies to elementary particles and their interactions, describing all their physical phenomena except gravity. General relativity explains the law of gravitation and its relation to other forces of nature. It applies to the cosmological and astrophysical realm, including astronomy.
The theory transformed theoretical physics and astronomy during the 20th century, superseding a 200-year-old theory of mechanics created primarily by Isaac Newton. It introduced concepts including spacetime as a unified entity of space and time, relativity of simultaneity, kinematic and gravitational time dilation, and length contraction. In the field of physics, relativity improved the science of elementary particles and their fundamental interactions, along with ushering in the nuclear age. With relativity, cosmology and astrophysics predicted extraordinary astronomical phenomena such as neutron stars,black holes, and gravitational waves.
Special relativity
Special relativity is a theory of the structure of spacetime. It was introduced in Einstein's 1905 paper "On the Electrodynamics of Moving Bodies" (for the contributions of many other physicists see History of special relativity). Special relativity is based on two postulates which are contradictory in classical mechanics:
- The laws of physics are the same for all observers in uniform motion relative to one another (principle of relativity).
- The speed of light in a vacuum is the same for all observers, regardless of their relative motion or of the motion of the light source.
The resultant theory copes with experiment better than classical mechanics. For instance, postulate 2 explains the results of the Michelson–Morley experiment. Moreover, the theory has many surprising and counterintuitive consequences. Some of these are:
- Relativity of simultaneity: Two events, simultaneous for one observer, may not be simultaneous for another observer if the observers are in relative motion.
- Time dilation: Moving clocks are measured to tick more slowly than an observer's "stationary" clock.
- Relativistic mass
- Length contraction: Objects are measured to be shortened in the direction that they are moving with respect to the observer.
- Mass–energy equivalence: E = mc2, energy and mass are equivalent and transmutable.
- Maximum speed is finite: No physical object, message or field line can travel faster than the speed of light in a vacuum.
- The effect of Gravity can only travel through space at the speed of light, not faster or instantaneously.
The defining feature of special relativity is the replacement of the Galilean transformations of classical mechanics by the Lorentz transformations. (See Maxwell's equations of electromagnetism).
General relativity
General relativity is a theory of gravitation developed by Einstein in the years 1907–1915. The development of general relativity began with the equivalence principle, under which the states of accelerated motion and being at rest in a gravitational field (for example, when standing on the surface of the Earth) are physically identical. The upshot of this is that free fall is inertial motion: an object in free fall is falling because that is how objects move when there is no force being exerted on them, instead of this being due to the force of gravity as is the case in classical mechanics. This is incompatible with classical mechanics and special relativity because in those theories inertially moving objects cannot accelerate with respect to each other, but objects in free fall do so. To resolve this difficulty Einstein first proposed that spacetime is curved. In 1915, he devised the Einstein field equations which relate the curvature of spacetime with the mass, energy, and any momentum within it.
Some of the consequences of general relativity are:
- Clocks run slower in deeper gravitational wells.. This is called gravitational time dilation.
- Orbits precess in a way unexpected in Newton's theory of gravity. (This has been observed in the orbit of Mercury and in binary pulsars).
- Rays of light bend in the presence of a gravitational field.
- Rotating masses "drag along" the spacetime around them; a phenomenon termed "frame-dragging".
- The universe is expanding, and the far parts of it are moving away from us faster than the speed of light.
Technically, general relativity is a theory of gravitation whose defining feature is its use of the Einstein field equations. The solutions of the field equations are metric tensors which define the topology of the spacetime and how objects move inertially.
Subscribe to:
Posts (Atom)








